RIVM assumes that something happened in 2021 that increased the mortality chances in the Netherlands, also for 2024. This can be seen from the figures for the increased mortality expectation (baseline), which also foresees higher exceedances. The cause is not named or explained.
The graph "Nationwide mortality" was temporarily provided by CBS during the corona period due to disagreements with the RIVM with regard to purpose and content. This shows that it is an important means of communication. The graph, as it is now presented by the RIVM, is accompanied by a (renewed?) public explanation. Some additional background knowledge is required to be able to understand what one wants to say, but above all: what it actually says. And what it doesn't say.
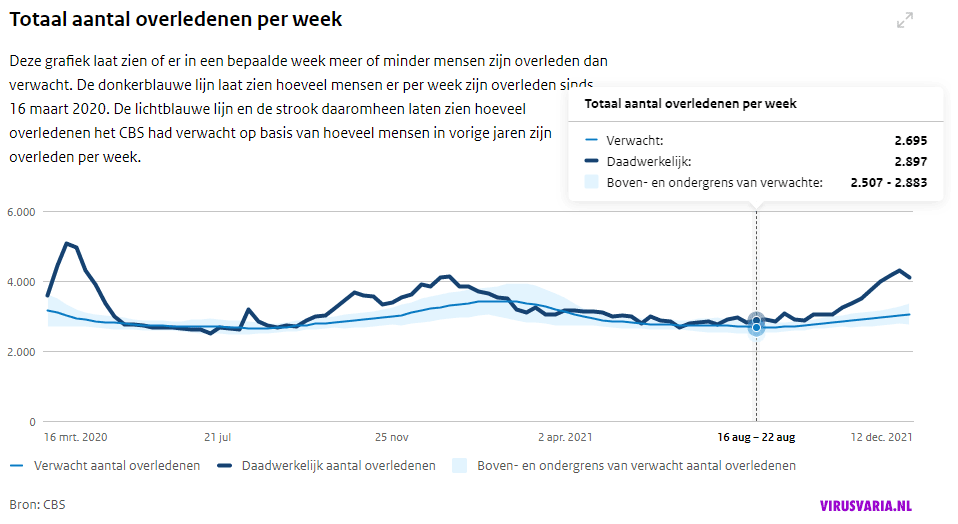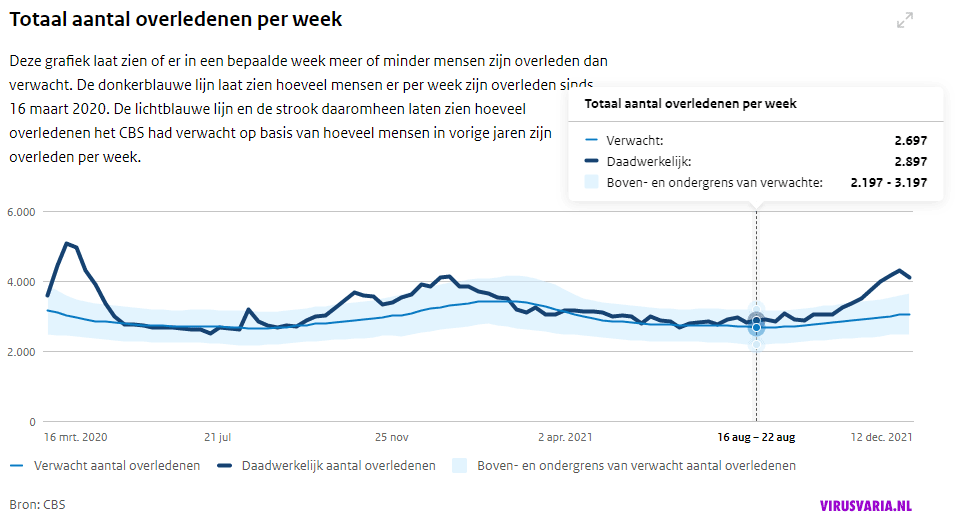
Just to be sure, I immortalized the text in a screenshot. You don't have to read it, I'll quote the relevant bits in the text.
The baseline is gone
The first thing you notice is that the RIVM graph no longer shows a baseline. It seems like a detail, but such a line does not disappear by accident, it is really thought about. The baseline is the expectation of the exact average for that particular week. Road designed.
With a baseline as a reference, you can immediately see whether the actual mortality is above or below the expectation for a particular week. If the actual mortality remains above baseline for six months or a year, it will no longer be possible to see it properly without a helpline.
As a reminder, an image of the old-style RIVM graphs: with baseline, the blue line in the middle of the bandwidth. I will now show you the picture of when the bandwidth was fiddled with. Completely accidental, of course, a conversion error that optically eliminated the excess mortality. (At the time, here signalled). That bandwidth is now being tampered with again.
Bandwidth utility: signal value
The new RIVM baseline with bandwidth is based on the past five years (excl. 2020), years in which a lot of misunderstood mortality occurred. These figures have been used to model a high mortality rate. Perhaps because a mortality prediction based on recent years is part of the job, while no one seems to know what such an estimate was actually for. Or they know something we don't know yet: excess mortality will continue for a while, get used to it.
The bandwidth always indicated the norm within which the mortality rates of a healthy population should move. Any excess of that bandwidth serves as an alarm signal. It indicates that there is unusually high mortality that week. A cause is then sought: usually a heat wave or a flu wave. A severe flu wave can last up to 10 weeks. By monitoring this properly, you keep an eye on public health and you can take appropriate action if necessary and also see when it goes away.
However, if a flu wave passes by and mortality nevertheless remains neatly within the bandwidth, then your prediction is of no use at all, then your band was too wide to use as a signal value. You only show that you have fulfilled your obligations: neatly predicted, even the flu wave falls within it.
- You don't install worn-out solar panels on your roof if you want to be environmentally conscious.
- You don't hang up fake smoke detectors to meet the fire safety requirements.
- You don't calculate a public health reference based on disastrous years.
Unless you know it's wrong, but you don't want to cause panic, for example, just to name a consideration. Then you start looking for ways to raise the standards so that the signal values are tapped less often. It seems so.
Bandwidth is King – but which one?
The National Institute for Public Health and the Environment (RIVM) explains what the bandwidth displays. The expected mortality is only mentioned as a tool to calculate the margin. The reasoning is that this expected mortality is based on the past five years – with the exception of 2020, because we know that there was a corona peak, so that does not count. But why not? The excess mortality years 2021 and 2022 (and soon also 2023) do count them and the excess mortality in those years was even higher than in 2020, looking at the seasonal years. Or take the past 10 years as a reference period, that would also dampen the effect of those crazy last excess mortality years.
The statisticians at CBS think otherwise. They also take 2021 and 2022 not in the prognosis, because these are clearly different years. According to them because of Covid, but then again, those are not medics.
So there are reasons for the agency of the Ministry of Health, the RIVM, to accept and 'normalize' that higher level of mortality as we have done with the winter season. If they wanted to mask excess mortality, they couldn't have done a better job.
Below is the RIVM explanation of the bandwidth.
The band represents the expected mortality between an upper and a lower limit. The upper limit is the expected mortality plus two standard deviations from the expected mortality. The lower limit is the expected mortality minus two standard deviations from the expected mortality.
https://www.rivm.nl/monitoring-sterftecijfers-nederland
There does not seem to be any policy behind this. Dry statistics. Let's calculate that using this week's RIVM download file.
This file also does not contain any expected mortality, only the actual weekly mortality and the upper and lower limit values for each week. The value that lies exactly in between is normally the expected value and that is for the last reported week: 3230.
"Two standard deviations of expected mortality". With a single value we speak of the 'uncertainty' and that is 2 times the square root of 3230 and that is: 114.
The upper limit would have to be something like: 3230 + 114 = 3344. But the upper limit specified by RIVM is no less than 95 higher: 3439, which is also consistent with the graph. The signal value is therefore much less sensitive with this upper limit, from 114 to 209.
This means that 95 more deaths must now occur in one week (85% more!) to achieve 'increased mortality' than the uncertainty of the weekly value itself. There may well be a reason for this, but what do we want now: to set a signal value to signal deviations from the expectation or do we want to normalize the excess mortality years, by including that increased mortality in the expectation?
It seems that the RIVM mainly wants to predict well.
The excess mortality is expected and thus disappears from the signalling.
Now, the "public explanation" on the RIVM website will undoubtedly be a simplification of the actual calculation method in which more uncertainties are included in one way or another, broadening the link somewhat. Choices also have to be made in statistics, and even "standard deviation" is a malleable concept: which period do you take, which peaks do you take into account or not. And if you don't include a peak, do you top it at the upper value or set it to zero: after all, mortality has been 'explained' and can be booked out of the forecast, just like a heat wave. But it is striking that there is a consistent upward trend.
We already knew that the baseline has been raised because the RIVM includes the years with unexplained excess mortality in the forecast, contrary to how CBS calculates. That in itself is worrying. You can expect the bandwidth to grow somewhat in absolute terms, as a percentage of the increased numbers. But that percentage itself doesn't increase from one year to the next, does it?
Well, it is. I'll show you, again using the same csv file. If we compare the first weeks of January, we see that the bandwidth (2x the standard deviation) increases compared to the expected mortality. The same goes for the first weeks of July.
| Upper limit as a percentage of expected mortality | ||
| Year | 1st week of January | 1st week of July |
| 2021 | 12.9% | |
| 2022 | 11.0% | 13.7% |
| 2023 | 11.8% | 14.1% |
| 2024 | 12.1% | |
Note: I do it percentage-wise, which is very lenient because the standard deviation becomes proportionally smaller with larger numbers. Carrot 4 = 2, which is 50%. Carrot 9 = 3, which is 33.3%. Carrot 16 = 4, which is 25%. The fact that the bandwidth, on the other hand, increases in percentage terms with higher numbers therefore requires some explanation.
Do you calculate it yourself?
The only thing that comes with it is addition, subtraction, division and multiplication. That's fun! 🙂
- First, calculate the baseline in a column (upper limit + lower limit divided by 2).
- In the column next to it, you do upper limit minus baseline. This in turn shows how many deaths still fall within the bandwidth of 1 week.
- If you divide that in the next column on the baseline, you have the percentage (you can see that it is not that difficult)
The upper limit in the 1st week of January 2022 was 11% higher than the expectation in that week. In 2024, the upper limit will have become 12.1%. From 11 to 12.1, that's a 10% increase in two years, on top of the already elevated baseline. Just by virtue of this seemingly minimal broadening of the band, any week 40 more people die without 'increased mortality' being detected.
In one year's time, that is also about 2,000 extra deaths. While the increase in the baseline already allowed approximately 9,000 additional deaths in 1 year, calculated from July 2023 to June 2024. (To calculate that, you also need traditional "virus varia baseline" that we use on sterftemonitor.nl, see also ResearchGate).
The total of additional 'unmarked excess mortality' will then amount to approximately 11,000 more deaths on an annual basis than before. These are numbers that make a flu epidemic pale in comparison and it is in the order of magnitude of corona year 2020, with two corona waves in 1 calendar year. In the future, a lot of excess mortality will escape the attention of the media.
So:
- The expectation has been raised
- With a higher expectation, the bandwidth should not even grow in percentage terms
- However, the bandwidth is becoming an increasingly large percentage of the expectation. The signal value is further increased, so that even more excess mortality goes unnoticed.
Could the bandwidth have been increased because there were so many peaks, and so much persistent excess mortality? Peaks are not taken into account, says the RIVM. If they only mean the part above the upper margin, there is still a lot of excess mortality between baseline and upper margin. And if it's based on real numbers, shouldn't the bottom margin be much smaller because there were virtually no under-mortality weeks in the last five years? Or will it be statistically modelled again? As the bottom margin is presented now, the only function is to mirror the top margin. That aside.
How important is that bandwidth?
The baseline is no longer used, everything is hung on the bandwidth. The light blue bandwidth is the safe area, exceeding it indicates "slightly elevated". In footnote 3, RIVM explains how we should see this gradually:
"If mortality is higher than 2 standard deviations above the expected mortality, we call mortality slightly elevated. At 3 standard deviations, we call mortality Increased. At 4 or more standard deviations, we call mortality greatly increased.”
https://www.rivm.nl/monitoring-sterftecijfers-nederland
What it doesn't say is that this signal value only applies to the week. For science columnists and spin doctors at CBS, this is a rewarding anchor that can be thrown out 52 times a year, but for people who can do a bit of math, it's different. If you take longer periods, the bandwidth will be smaller (also think of the Carrot calculation). Yet, week after week, RIVM and CBS are once again looking exclusively at the last week only. Compare it to flipping a coin. Free to an earlier post Bandwidths for dummies:
If you flip the coin 28 times and you get, for example, 24 heads or 24 tails, that is a signal that something is wrong with your coin (that chance is 0.0153%, you would have to roll about 700,000 to encounter such a sequence). Then you will either have to adjust your expectations regarding this coin or reject the coin and start investigating. If you still stubbornly insist "yes, but every time I threw it was really a one in two chance so there is nothing special to see" then you apply the wrong rule and close your eyes to the series you are making.
RIVM and CBS consider each week to be isolated, while, for example, since October 2023 until now (32 weeks), there was only a mortality rate in 4 weeks that was lower than the (increased) expectation, and higher in 28 weeks. That's the orange line. If we look at the excess mortality based on the traditional expectation (grey line in the graph), as calculated before 2024, the mortality has not fallen below baseline once since July 2023. An uninterrupted excess mortality series of approximately 40 weeks: significant excess mortality.
We therefore do not know exactly what RIVM means by a "standard deviation" to be able to make calculations. In any case, for them, it is something that grows as more people die, just like the baseline made invisible. It is a mix between hard statistics (the lower margin that is the same as the upper margin) and on the other hand it is attributed to reality because of the increased exceedances at the top. And then the bottom goes along again, of course, because that's how it should be with statistical uncertainty margins...
Where are we now?
See the orange line for 2024. The X-axis of this graph (thanks to Herman Steigstra) goes from August to July. Seasonal years give a clearer picture because the winter bumps are not intersected by the turn of the year. In '22-'23 (green line) there was slightly less excess mortality than in '21-'22 and now we are slightly lower again. But still far above the corona season '19-'20. And also above '20-'21, when everyone was vaccinated.
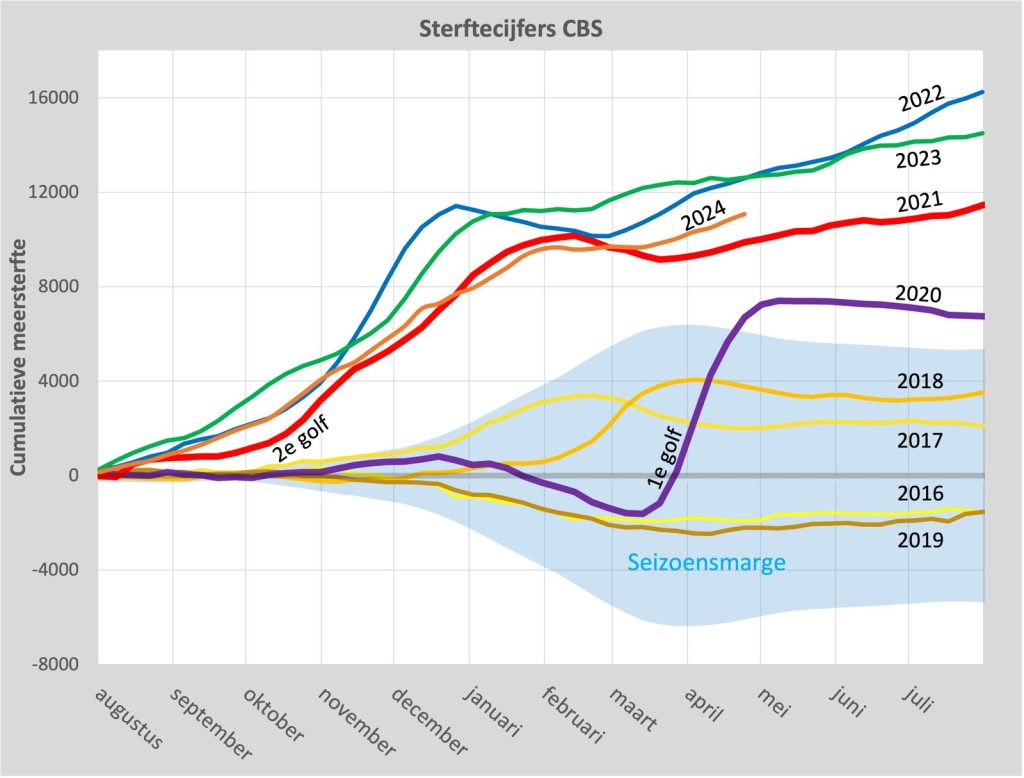
What I find particularly disgusting about this graph: Before 2021, for example in the summer after the 1st wave, the line decreases again (under-mortality) after the excess mortality period. You can also see that after the bumps in 2017 and 2018: a small decrease and then stabilization.
But then, in 2021, 2022 and 2023, it just continues to rise in the summer. That really doesn't sit well. The fact that no attention is paid to this means to me that there is nothing they can do about it. It is accepted, a fait accompli. And it's going to happen again. If that has anything to do with policy, then there is no other option than to insist that they have done the right thing and simply advise pregnant women to take the jab again. Otherwise, there will be a cognitive dissonance that cannot be managed.
Looking further ahead
If mortality slowly returns to normal in the coming years, the RIVM will report under-mortality until it becomes too much and they will correct the baseline again because things are going so well. In any case, a lot of excess mortality fuss has been missed. Let's keep our fingers crossed that we don't have to wait years for that and that we will then also be back in line with pre-2020 levels. For the time being, this graph offers little hope of such an improvement.
In the Previous article we saw that the average age of death in 2021 and 2022 was five years lower than before.
If we are to believe some doomsday scenarios (Vandenbossche, Dowd, Cole, McCullough, McKernan, Bonte, etc.), the mortality level could remain higher for decades. That remains to be seen. By raising the baseline, RIVM has at least safely prepared for this. They do this for a reason: they already know it, as can be seen from the figures.
Questions about the techniques used to identify less excess mortality:
- Why does RIVM use different comparison years than CBS, resulting in a higher mortality expectation (baseline)?
- What is the justification for the expanded margin of uncertainty, which means that the signal value for excess mortality is further from the baseline?
- This margin is stretched a little further every year, more than what can be expected on the basis of the baseline. Why this step-by-step increase in tolerance, always in July?
- Weeks were not assessed by CBS as a series but as isolated events, which meant that long-term excess mortality was not detected. In the health sector, taking history into account is necessary for a diagnosis. Will RIVM continue to look exclusively at exceedances of the weekly margin?
- The National Institute for Public Health and the Environment (RIVM) does not show a baseline in the graph, which makes it difficult to visually identify excess mortality and excess mortality (especially over several weeks). Can RIVM bring that line back and if not, what is the consideration for this?
The National Institute for Public Health and the Environment, as an agency of the Ministry of Health, Welfare and Sport, will not comment on where the years of excess mortality came from, tens of thousands of people who died. The expectation was correct, will then be the observation.
If that isn't worth an honorary doctorate or scientific medal of exceptional merit, I don't know what is.


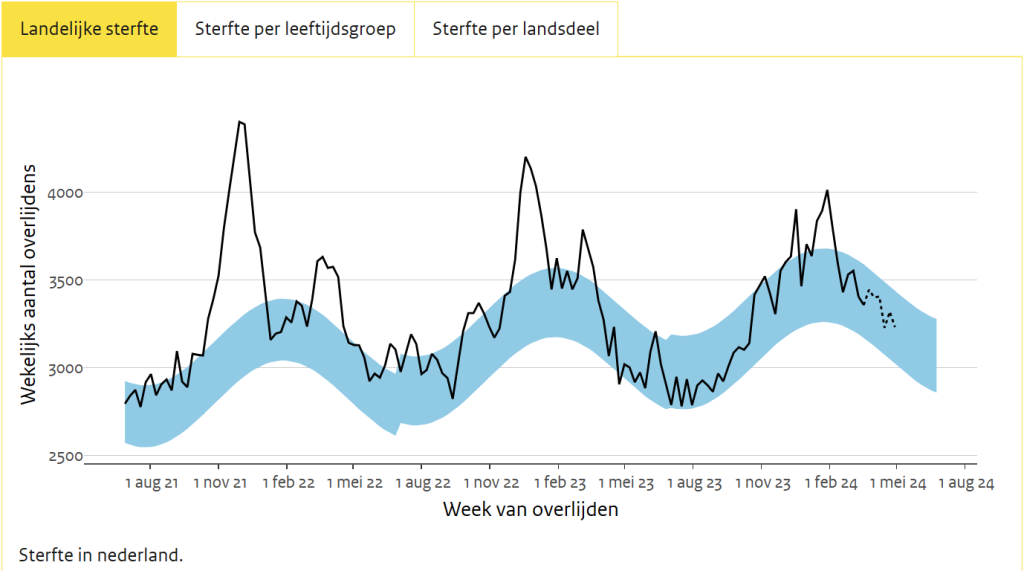



Would the RIVM keep shadow accounts? Because, of course, they will miss flu and heat waves (or only signal their peaks).
Until Dec. 2023, the RIVM stated this on the page https://www.rivm.nl/monitoring-sterftecijfers-nederland :
“Sinds de griepepidemie van 2009 houdt het RIVM wekelijks het aantal overleden mensen in de gaten met gegevens van het CBS. Het doel van het monitoren van sterftecijfers is om de impact van een epidemie of een incident in beeld brengen. In het verleden zijn er pieken in de totale sterfte te zien bij koude- en hittegolven en bij uitbraken van infectieziekten, zoals griep.
De oversterfteschattingen van het CBS en het RIVM kunnen op weekniveau verschillen.
⦁ Het CBS kijkt naar de gemiddelden over de afgelopen jaren voor de betreffende week, deze zijn inclusief verhoogde sterfte in het griepseizoen.
⦁ Het RIVM wil juist ook elk jaar oversterfte door de griep in kaart brengen. Om die reden verschillen de schattingen van het CBS en van het RIVM. ”
Nu stelt zij:
“Het RIVM en het CBS houden sinds 2009 elke week het aantal overleden mensen in Nederland bij. Hiermee kunnen de gevolgen van bijzondere omstandigheden op het aantal overleden mensen in beeld gebracht worden. Bijvoorbeeld bij een hitte- of koudegolf, een griepgolf of de uitbraak van een infectieziekte. “
En heel veel dank, Anton, voor de signalering en de uitleg. Het is zeer wrang dat dit in de “gewone media” niet eens opgemerkt werd en wordt.
inmiddels meer onderzoek: oversterfte moet onderzocht want lijkt te komen door de prikken:
“Conclusions Excess mortality has remained high in the Western World for three consecutive years, despite the implementation of containment measures and COVID-19 vaccines. This raises serious concerns. Government leaders and policymakers need to thoroughly investigate underlying causes of persistent excess mortality.”
https://bmjpublichealth.bmj.com/content/2/1/e000282
Ja er komen er steeds meer. Ik zal binnenkort een aantal verzamelen en in een artikel op een rijtje zetten.
I only see this now, very interesting, thank you! But I am puzzled by the claim that "the 'uncertainty' [...] is 2 times the square root of 3230". I haven't seen anything like it before.
Usually, the standard uncertainty (SD) is based on the deviations of the mean – and not the square root of the mean. If the data is put into Excel or Openoffice, the standard deviation can easily be calculated. However, the use by RIVM is more vague, because they do not include (or take?) flu waves. For example, in the . CSV for May to September 2023 with =STDEV(B98:B119) a flu-free sample standard deviation of 103.6, so two SD is 207. The RIVM takes, if I understand correctly, (D141-C141)= 419 as the bandwidth for 2024, which is two times two SD so that 2*SD=209.5 and I don't think there's anything wrong with that.
In times with more fluctuation, the standard celebration is greater than before, by definition. But whether that should be considered "normal" is of course also a point that can be debated. I don't think so, with the same arguments as why the excess mortality that has now become "normal" should not be considered "normal".
'Whether that should be considered "normal" is of course also a point that can be debated.' That is indeed the most important point. See also the Broadening margins.
The forecast of RIVM, which is now visible up to and including wk 43 (end of October), shows a remarkably steeper curve from wk 35 onwards than in previous years. The expected "autumn bumps" will remain nicely in between, but the peaks of heat waves will still be visible.